Introduction

PLEASE run the orbit simulator for the object of interest and print out the plot of its orbit before using this calculator. Data measured from the print-out will be necessary for the calculations below.

I. Kepler's First Law of Planetary Motion

Planets orbit the Sun in ellipses with the Sun at one focus of the ellipse.

Introduction to Orbital Shape

The eccentricity of an orbit is a measure of how elongated it is, that is, how non-circular it is. To comprehend this more clearly, students will measure the orbit on the print-out and then calculate the eccentricity from these measurements. These results will then be compared with the original value.

Calculating the Eccentricity
  1. Find the length of the semi-major axis of the orbit (a) in cm. Use a ruler to draw a straight line through the longest dimension of the plot of the orbit. (This line will go through the location of the Sun.) This is the major axis. Measure the length of this line from where it touches one end of the orbit to where it touches the other end of the orbit. Divide this value by 2 to find the length of the semi-major axis.
  2. Find the length of the semi-minor axis of the orbit (b) in cm. Locate the center of the major axis and use a protractor to draw a perpendicular line through this point from one side of the orbit to the other side. This is the minor axis. Measure the length of this line. Divide this value by 2 to find the length of the semi-minor axis.
  3. Locate the other focus of the orbit. An ellipse has two foci. One is where the Sun is located and is indicated by the '+' mark near the center of the printed orbit. The other focus is also on the major axis and is located equal distance from the minor axis opposite to the first focus. Measure accordingly with your ruler and mark the second focus.
  4. Measure the distance between the two foci of the orbit in cm.
  5. Enter the above data into the appropriate spaces in the equations below.

One formula is:

  e2    =    1 - ( b2 / a2 )
  ( )2 = 1 - [( cm )2 / ( cm )2]
(Press the calculate button to find the eccentricity of the plotted orbit.)

The second formula is:

  e    =    (distance between the foci) / ( 2 a )
  = cm / ( 2 X cm )
(Press the calculate button to find the eccentricity of the plotted orbit.)

Compare these two values together and with the eccentricity used to plot the orbit. They should agree to the number of significant digits used to make the appropriate measurements from the print-out.

II. Kepler's Second Law of Planetary Motion

Planets move in their orbits at a rate such that a line connecting the planet and the Sun will sweep through equal areas in equal time intervals.

Introduction to Orbital Velocities

Kepler's Second Law only gives a relationship between the velocities of an orbiting object at two points in its orbit and not a way to calculate any particular velocity. Isaac Newton, with his more theoretical understanding of the motion of an object, his formulation of the force of gravity and his concept of the conservation of angular momentum, was able to derive a formula for the velocity of an orbiting object.

The sketch at left shows a visual representation of Kepler's Second Law. Each sector is diagrammed for the same length of time as the object moves in its orbit. Notice how the object is moving slower in its orbit when it is furtherest from the Sun.

 A sketch of equal area sectors radiating from the Sun.
Calculating Orbital Velocities

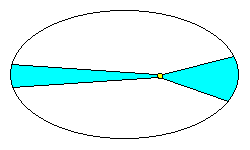
Confirm that the information already given at the top of this web page has been correctly entered in the appropriate boxes below, where:

The constants in the following equations have been chosen so as to give the orbital velocity of the object in m/s.

Orbital velocity at aphelion (point in orbit furtherest from the Sun):

  va2    =    ( 8.871 X 108 / a ) ( 1 - e ) / ( 1 + e )
  ( m/s )2 = ( 8.871 X 108 AU . m/s / (Press the calculate button to find the velocity of the object at aphelion.)

Orbital velocity at perihelion (point in orbit closest to the Sun):

  vp2    =    ( 8.871 X 108 / a ) ( 1 + e ) / ( 1 - e )
  ( m/s )2 = ( 8.871 X 108 AU . m/s / (Press the calculate button to find the velocity of the object at perihelion.)
III. Kepler's Third Law of Planetary Motion

If the orbital period is given in years and the semi-major axis of the orbit is given in AU, then the period squared is equal to the semi-major axis cubed.

Introduction to Orbital Periods

Kepler formulated his conclusion of how the period of an object orbiting the Sun is related to the average distance the object is from the Sun into his Third Law. The average distance an object is from the Sun is equal to the semi-major axis of its orbit. Again Newton was able to extend Kepler's concept. Newton showed that this relationship also depended upon the sum of mass of the object and the mass of the Sun. However, since Jupiter, the largest planet is only about 0.1% of the mass of the Sun, this additional variable is not significant at the level of three significant digits.

Calculating the Orbital Period

Confirm that the length of the semi-major axis given at the top of this web page has been correctly entered below, where:

With these units, Kepler's Third Law is simply:

  p2    =    a3
  ( yr )2 = ( (Press the calculate button to find the orbital period of the object.)